There are some dimensionless numbers in chemical engineering which are important to process engineer‘s daily routine. These numbers help engineers analyze various problems more quickly. They are also calculated as a precursor to mostly used physical quantities such as overall heat transfer coefficient and pressure drop.

Engineering problems are categorized into three major parts called transport phenomena. Heat, mass and momentum transfer are utilized to describe almost any chemical engineering problem. Therefore, it is much easier if we can deal with all of them similarly. It’s also necessary that our calculations are independent of any system of units. As a results, these dimensionless numbers in chemical engineering play an important role in gas and oil industry. In the following section, we will review some of the most important dimensionless quantities with their practical usages.

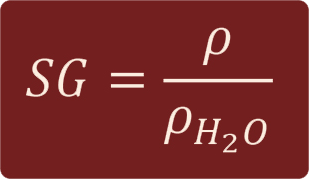
specific gravity - dimensionless numbers

Specific gravity illustrates how heavy (dense) a fluid is, compare to water at 4 °C. Normally for all component, specific gravity decreases with the rise in temperature. Petroleum API number is defined based on specific gravity of the oil sample. The less the specific gravity, the higher the API number, thus the more valuable the oil would be. (In this equation rho=density)

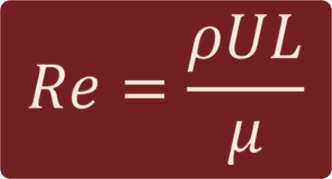
reynold - dimensionless numbers

Reynold number is by far the most famous dimensionless number. It is the ratio of fluid inertia to viscous force. Main applications are to determine the coefficient of friction and to specify either a flow is laminar or turbulent. Fluid velocity is the most important parameter in this equation. As a rule of thumb, the higher the velocity the higher the Reynolds number, thus the higher the friction factor. (In this equation rho=density, U=velocity, L=equivalent diameter, mu=viscosity)

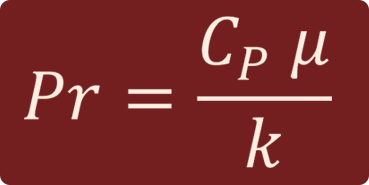
prandtl - dimensionless quantities

Prandtl number which is used in heat transfer applications is the ratio of viscous to thermal diffusivity. Mainly used to calculate heat transfer coefficient. As a rule of thumb, The higher the Prandtl number the higher the heat transfer coefficient. Prandtl number is only a function of fluid’s physical properties. (In this equation Cp=specific heat, mu=viscosity, k=thermal conductivity)

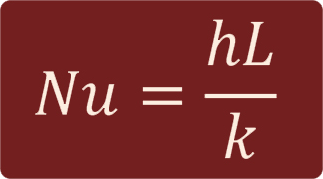
nusselt - dimensionless quantities

Nusselt number which is used in heat transfer applications is utilized to determine convective heat transfer coefficient and as a result, overall exchanger heat transfer coefficient. The more the Nusselt number, the higher the heat transfer rate. Most of the heat exchanger’s calculations is based on finding this value. Normally, Nusselt is a direct function of  Reynold & Prandtl. (In this equation h=convective heat transfer coefficient, L=characteristic length of heat transfer film layer, k=thermal conductivity)

schmidt - dimensionless numbers

Schmidt number, similar to Prandtl number, is used in mass transfer applications. It is the ratio of viscous to molecular diffusivity. Mainly used to calculate mass transfer coefficient. As a rule of thumb, the higher the Schmidt number, the higher the mass transfer coefficient. Schmidt number is only a function of fluid’s physical properties. (In this equation mu=viscosity, rho=density, D=mass diffusivity)

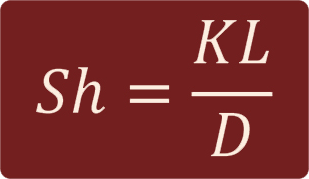
sherwood - dimensionless numbers

Sherwood number, similar to Nusselt number, is used in mass transfer applications. It is utilized to determine mass transfer coefficient. The more the Sherwood number, the higher the mass transfer rate. Normally, Sherwood is a direct function of Reynold & Schmidt. This value plays an important role in designing of distillation columns, absorber, scrubber, etc.  (In this equation K=convective mass transfer coefficient, L=characteristic length of mass transfer film layer, D=mass diffusivity)